In the valuable paper on "American Railroad Bridges," read by Mr. THEODORE COOPER at the Annual Convention of the American Society of Civil Engineers, an abstract from which was reprinted in ENGINEERING NEWS of July 6, the lack of accuracy in using equivalent uniform loads in place of the actual wheel concentrations is clearly demonstrated; and the analytical method in common use for determining the maximum shears and moments for the actual wheel concentrations is succinctly stated. This method is also fully explained in BURR'S "Stresses in Bridge and Roof Trusses," edition of 1888.

The advantages, as shown by Mr. COOPER, in using the actual wheel concentrations is not that the individual strains may be found with mathematical accuracy, but that the correct relative proportion of the several parts of the bridge is thus secured, for the assumed loading.

The objection to the use of typical train loads is the labor involved in treating the load on each wheel separately. This labor may be greatly reduced by the analytical method given by Mr. COOPER; but even with this method, considerable arithmetical work is necessary.

Now the desideratum being to find with the least labor the correct relative proportions of the several parts, and the strains on the individual members, to a close but not absolute degree of accuracy, it would appear that the graphical method is peculiarly adapted to this purpose.

It has been the custom of the writer to use a graphical diagram for ascertaining the maximum strains in bridges, and this method has been found sufficiently accurate for all practical purposes, reducing the liability to error and the arithmetical work to a minimum.

The diagram for any given load may be made in a few moments on a piece of profile paper as follows:
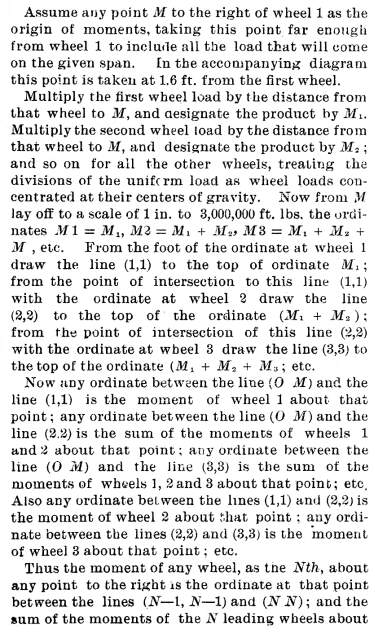
At the top lay off to a scale of 1-16 in. to 1 ft. the distances between the wheels, and the distances center to center of gravity of short lengths of the uniform train load, and draw ordinates through these points. On these ordinates lay off from the line (O M) at the bottom of the sheet the sums of the loads up to and including the load at each ordinate, and draw horizontal lines from these points to the following ordinates. The stepped line thus formed may be designated as the load line.

then, is the manner of finding the position of the loading that will produce a maximum moment at any point of a simple girder or truss: Place the slip of paper A, B, on which the span is marked, with the point e, at which the maximum moment is required, under any assumed wheel, and stretch a fine thread from a to the point of intersection of a vertical through B with the load line. If this thread cuts the part of the load line representing the wheel over c, then the loading produces a maximum moment at c. If the thread passes above this part of the load line, move the loading to the left: if it passes below, move the loading to the right.

It is thus seen that with the use of the load line no arithmetical work is necessary to ascertain the positions of the loading producing maximum moments.

Now for the special case above, the assumed position of the loading with wheel 4 over c produces a maximum moment at c, since the thread crosses the part of the load line representing wheel load 4.

Diagram for Concentrated Rolling Load